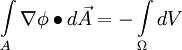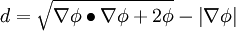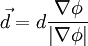Transport equation based wall distance calculation

From CFD-Wiki

Wall-distance variable

Wall distance are required for the implementation of various turbulence models. The approaximate values of wall distance could be obtained by solving a transport equation for a variable called wall-distance variable or 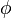$\phi$ .
The transport equation for the wall distance variable could be written as:

$\int\limits_A {\nabla \phi \bullet d\vec A} = - \int\limits_\Omega {dV}$

with the boundary conditions of Dirichlet at the walls as $\phi = 0$ and Neumann at other boundaries as ${{\partial \phi } \over {\partial n}} = 0$

This transport equation could be solved with any of the approaches similar to that of Poisson's equation.

Wall distance calculation

Wall distance from the solution of this transport equation could be easily obtained as:

$d = \sqrt {\nabla \phi \bullet \nabla \phi + 2\phi } - \left| {\nabla \phi } \right|$

Where as the wall distance vector could be written as:

$\vec d = d{{\nabla \phi } \over {\left| {\nabla \phi } \right|}}$

The foregoing method for evaluation of wall distances was proposed by D.B.Spalding [1994], mainly for the purpose of calculating, for arbitrary complex geometries, the wall distances required by various low-Reynolds-number turbulence closure models. Spalding's proposal was mainly intuitive, but the method has been described in a formal mathematical framework by Fares & Schroder (2002).

D.B.Spalding, ‘Calculation of turbulent heat transfer in cluttered spaces’, Proc. 10th Int. Heat Transfer Conference, Brighton, UK, (1994).

E.Fares & W.Schroder, ‘Differential Equation for Approximate Wall Distance' Int.J.Numer.Meth., 39:743-762, (2002).

Return to Numerical Methods

Transport equation based wall distance calculation

From CFD-Wiki

Wall-distance variable

Wall distance calculation

Views

My wiki

wiki navigation

wiki search

wiki toolbox