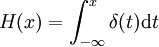Heaviside function
From CFD-Wiki
The Heaviside step function, H, also called the unit step function, is a discontinuous function whose value is zero for negative argument and one for positive argument.
It seldom matters what value is used for H(0), since 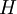 is mostly used as a distribution. Some common choices can be seen below.
is mostly used as a distribution. Some common choices can be seen below.
The function is used in the mathematics of control theory and signal processing to represent a signal that switches on at a specified time and stays switched on indefinitely. It was named in honor of the English polymath Oliver Heaviside.
It is the cumulative distribution function of a random variable which is almost surely 0. (See constant random variable.)
The Heaviside function is an antiderivative of the Dirac delta function: H′ = δ. This is sometimes written as
although this expansion may not hold (or even make sense) for x = 0, depending on which formalism one uses to give meaning to integrals involving δ.