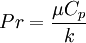Prandtl number
From CFD-Wiki
(Difference between revisions)
(changed from = to \equiv) |
|||
| Line 2: | Line 2: | ||
:<math> | :<math> | ||
| - | Pr | + | Pr = \frac{\mu C_p}{k} |
</math> | </math> | ||
| Line 10: | Line 10: | ||
* <math>C_p</math> is the specific heat at constant pressure | * <math>C_p</math> is the specific heat at constant pressure | ||
* <math>k</math> is the coefficient of thermal conduction | * <math>k</math> is the coefficient of thermal conduction | ||
| + | |||
| + | |||
| + | It is the ratio of momentum diffusivity (kinematic viscosity) to thermal diffusivity. It can be related to the thickness of the thermal and velocity boundary layers. It is actually the ratio of velocity boundary layer to thermal boundary layer. When Pr=1, the boundary layers coincide. When Pr is small, it means that heat diffuses very quickly compared to the velocity (momentum). This means the thickness of the thermal boundary layer is much bigger than the velocity boundary layer for liquid metals. | ||
| + | |||
| + | |||
[[Category: Dimensionless parameters]] | [[Category: Dimensionless parameters]] | ||
Revision as of 06:03, 20 December 2005
The Prandtl number is defined as
where
-
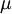 is the dynamic viscosity coefficient
is the dynamic viscosity coefficient
-
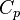 is the specific heat at constant pressure
is the specific heat at constant pressure
-
 is the coefficient of thermal conduction
is the coefficient of thermal conduction
It is the ratio of momentum diffusivity (kinematic viscosity) to thermal diffusivity. It can be related to the thickness of the thermal and velocity boundary layers. It is actually the ratio of velocity boundary layer to thermal boundary layer. When Pr=1, the boundary layers coincide. When Pr is small, it means that heat diffuses very quickly compared to the velocity (momentum). This means the thickness of the thermal boundary layer is much bigger than the velocity boundary layer for liquid metals.