Incompressible flow

From CFD-Wiki

(Difference between revisions)

Revision as of 08:44, 12 September 2005

A flow is said to be incompressible if the density of a fluid element does not change during its motion. It is a property of the flow and not of the fluid. The rate of change of density of a material fluid element is given by the material derivative

$\frac{D \rho}{D t} = \frac{\partial \rho}{\partial t} + u_j \frac{\partial \rho}{\partial x_j}$

From the continuity equation we have

$\frac{D \rho}{D t} = - \rho \frac{\partial u_j}{\partial x_j}$

Hence the flow is incompressible if the divergence of the velocity field is identically zero. Note that the density field need not be uniform in an incompressible flow. All that is required is that the density of a fluid element should not change in time as it moves through space. For example, flow in the ocean can be considered to be incompressible even though the density of water is not uniform due to stratification.

Governing Equations

The Navier Stokes equations for incompressible flow are

Continuity equation

$\frac{\partial u_j}{\partial x_j} = 0$

Momentum equation

$\frac{\partial u_i}{\partial t} + u_j \frac{\partial u_i}{\partial x_j} + \frac{1}{\rho} \frac{\partial p}{\partial x_i} = \nu \Delta u_i$

Energy equation

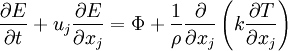
$\frac{\partial E}{\partial t} + u_j \frac{\partial E}{\partial x_j} = \Phi + \frac{1}{\rho} \frac{\partial}{\partial x_j} \left( k \frac{\partial T}{\partial x_j} \right)$

where

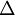$\Delta$ is the Laplacian operator
E is the internal energy per unit mass
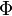$\Phi$ is the rate of dissipation of mechanical energy per unit mass

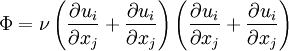
$\Phi = \nu \left( \frac{\partial u_i}{\partial x_j} + \frac{\partial u_i}{\partial x_j} \right) \left( \frac{\partial u_i}{\partial x_j} + \frac{\partial u_i}{\partial x_j} \right)$

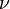$\nu$ is the kinematic viscosity
k is the coefficient of thermal conductivity
T is the temperature

Physical characteristics

A consequence of incompressible flow is that there is no equation of state for pressure unlike in compressible flow. Since there is no separate equation for pressure it must be obtained from the continuity and momentum equations. The main role of pressure is to satisfy the zero divergence condition of the velocity field. Note that pressure is only determined upto a constant.

If the viscosity is assumed to be constant then the energy equation is decoupled from the continuity and momentum equations.

@@ Line 49: / Line 49: @@
 * k is the coefficient of thermal conductivity
 * T is the temperature
+== Physical characteristics ==
+A consequence of incompressible flow is that there is no equation of state for pressure unlike in compressible flow. Since there is no separate equation for pressure it must be obtained from the continuity and momentum equations. The main role of pressure is to satisfy the zero divergence condition of the velocity field. Note that pressure is only determined upto a constant.
+If the viscosity is assumed to be constant then the energy equation is decoupled from the continuity and momentum equations.

Incompressible flow

From CFD-Wiki

Revision as of 08:44, 12 September 2005

Governing Equations

Physical characteristics

Views

My wiki

wiki navigation

wiki search

wiki toolbox